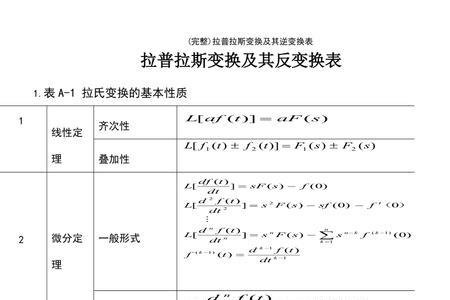
Laplace变换公式推导:
L[f(x)]=∫f(x)e^(-st)dt。拉普拉斯逆变换为当已知信号函数x(t)的拉普拉斯变换X(s),求解信号的时域表达式x(t)。
F(p)=int_0^infty e^{-pt}f(t)dt,quad p=s+isigma 记为: F(p)=mathscr{L}{f(t)},f(t)=mathscr{L}^{-1}{F(p)} 或写为: F(p)risingdotseq f(t),f(t)fallingdotseq F(p)

原创 | 2022-11-19 07:31:13 |浏览:1.6万
Laplace变换公式推导:
L[f(x)]=∫f(x)e^(-st)dt。拉普拉斯逆变换为当已知信号函数x(t)的拉普拉斯变换X(s),求解信号的时域表达式x(t)。
F(p)=int_0^infty e^{-pt}f(t)dt,quad p=s+isigma 记为: F(p)=mathscr{L}{f(t)},f(t)=mathscr{L}^{-1}{F(p)} 或写为: F(p)risingdotseq f(t),f(t)fallingdotseq F(p)



Copyright 2005-2020 www.kxting.com 版权所有 |  湘ICP备2023022655号
湘ICP备2023022655号
声明: 本站所有内容均只可用于学习参考,信息与图片素材来源于互联网,如内容侵权与违规,请与本站联系,将在三个工作日内处理,联系邮箱:47085,1089@qq.com